Play with POV-Ray
The following gif animation describes a shearing (twisting, or real earthquake) deformation of quasi-Fuchsian groups fixing bending angles. (Click the image if you want to see a higher resolution.)
The next is a bending deformation.
These describe deformations of quasi-Fuchsian groups of a genus 2 surface. We can take three non-separating simple closed curves (disjoint, non-homotopic each other) on the surface, which give a pants decomposition. In the above pictures, the hyperbolic lengths of these curves are fixed to be 2. In the first one, we also fix the bending angles (about π / 2) along these curves, but vary the twisting parameters. In the second picture, we fix the twisting parameters to be the halves of the lengths of these simple closed curves, and vary the bending angles instead.
Since these hyperbolic structures have symmetry of order 3, the deformation space is essentially equivalent to the one of a sphere with four cone points of angle 2π / 3.
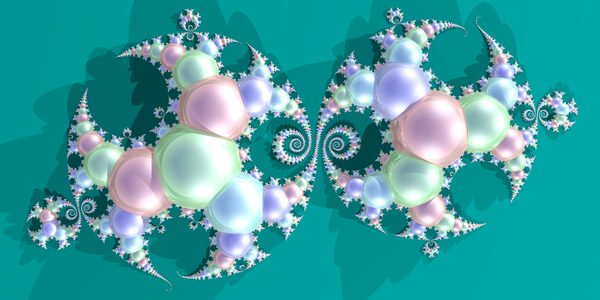
The next is an example from a genus 2 surface.
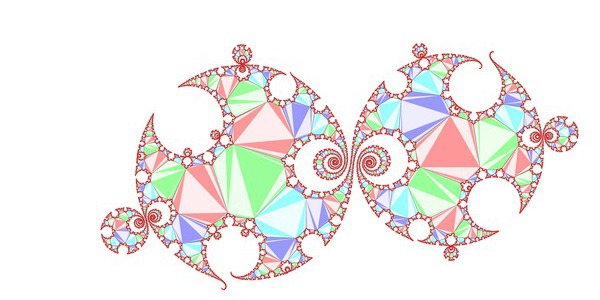
The image created by povray looks beautiful, but 2 dimensional picture is more informative.
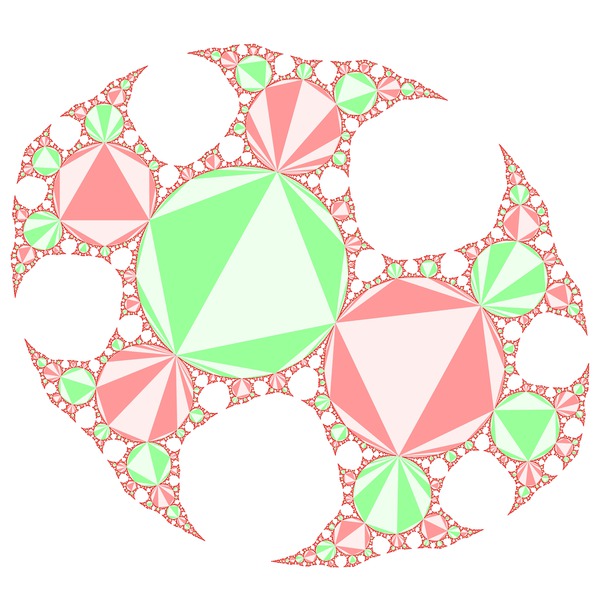
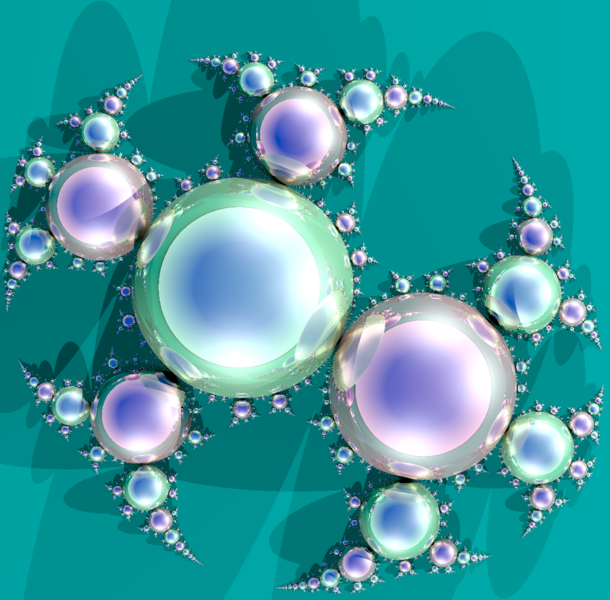
The next pictures are from a genus 3 surface.