Deformation of quasi-Fuchsian groups
The following indicates a deformation of a quasi-Fuchsian group of genus 3. The genus three surface is decomposed into 4 three-holed spheres (a.k.a. pairs of pants) corresponding to red, green, blue, and light blue in the picture below.
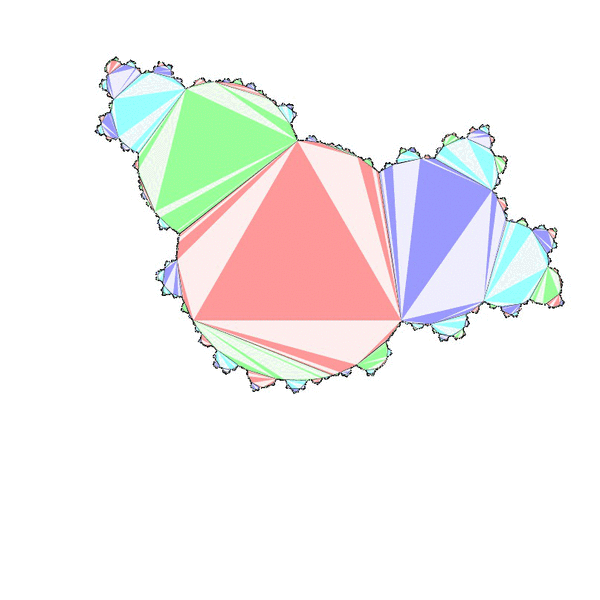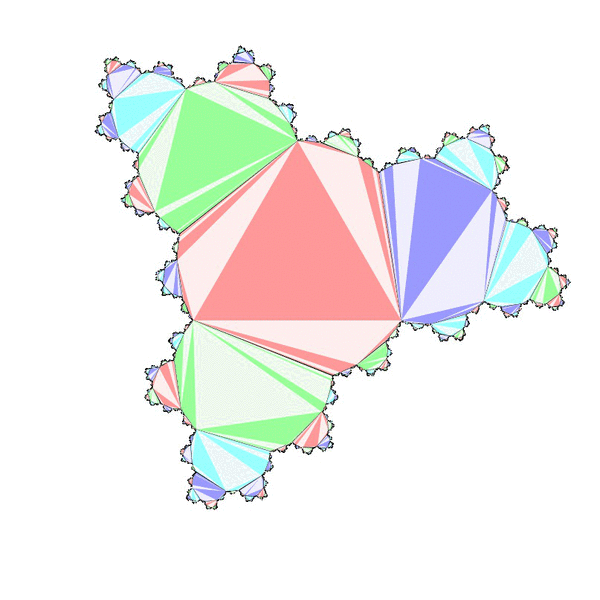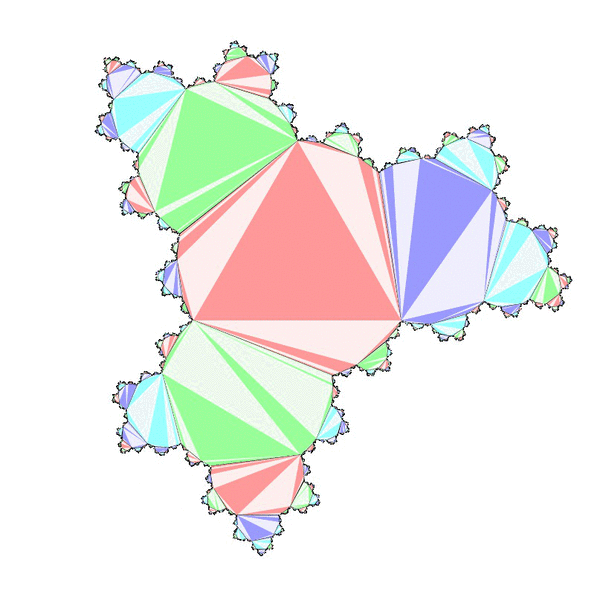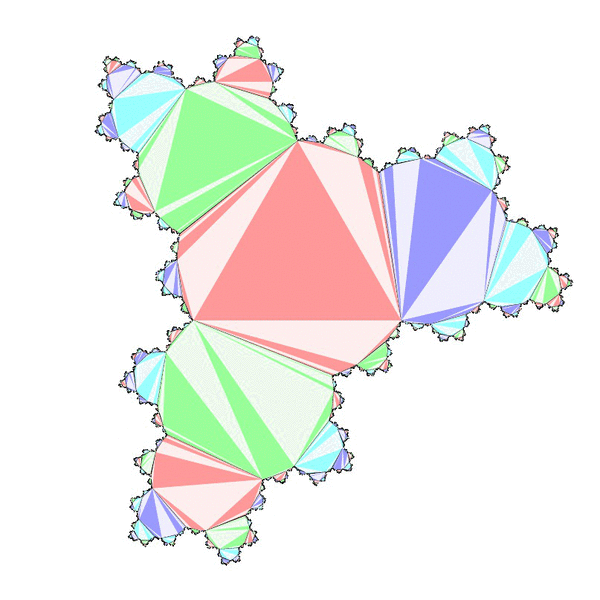
This animation describes a shearing-bending deformation about the simple closed curve shared by red and green pairs of pants. This and the following pictures are produced by my computer program based on the paper "Parametrization of PSL(2,C)-representations of surface groups".
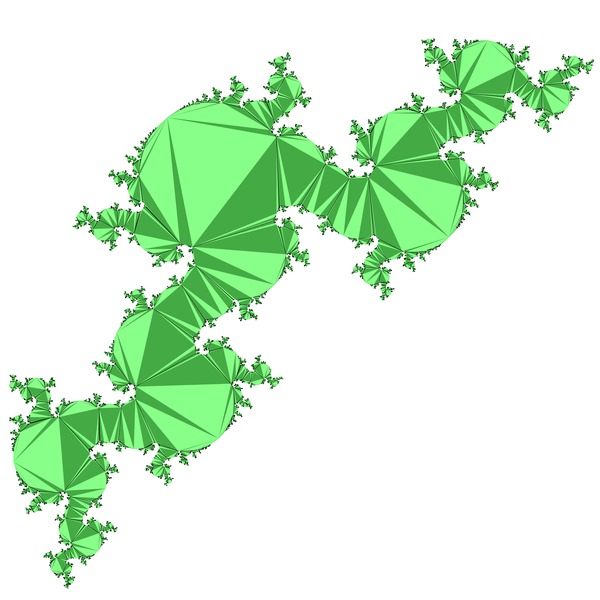
The next is a quasi-Fuchsian group of a surface of genus 2, which is obtained from a Fuchsian group bent along three simple closed curves.
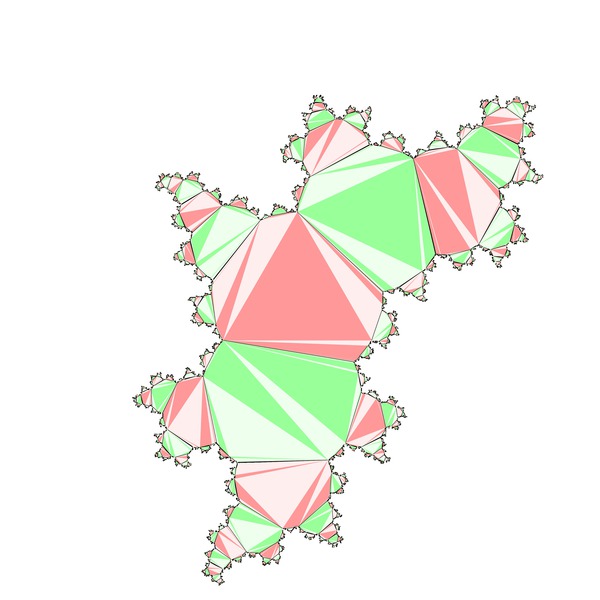
The next is a once-punctured torus group.
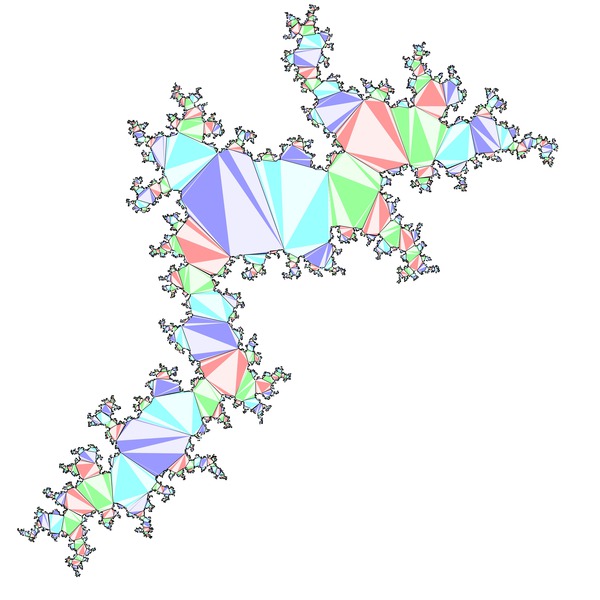
A more complicated example from a genus 3 surface.
I am interested in the domain of discrete faithful representations in the character variety, in particular, the boundary of it, action of the mapping class group on it, etc. At present, my computer program is not sufficient to study these objects, but does work well to draw above pictures.